|
Share this page: |
|
|
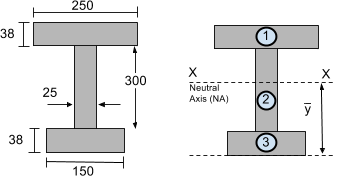
How to Calculate the Moment of Inertia of a Beam Section Before finding the moment of inertia (or moment of area) of a beam section, its centroid (or center of mass) must be known. For instance, if the moment of inertia of the section about its horizontal (XX) axis was required then the vertical (y) centroid would be needed first (Please view our Tutorial on how to calculate the Centroid of a Beam Section). Consider the I-beam section below, which was also featured in our Centroid Tutorial. As shown below the section was split into 3 segments:
The Neutral Axis (NA) or the horizontal XX axis is located at the centroid or center of mass. In our Centroid Tutorial, the centroid of this section was previously found to be 216.29 mm from the bottom of the section. Now to calculate the total moment of ienrtia of the section we need to use the "Parallel Axis Theorem": It is widely known that the moment of inertia of a rectangular area about its centroid axis is simply: Now we have all the information we need to use the "Parallel Axis Theorem" and find the total moment of inertia of the I-beam section: Of course you don't need to do all these calculations manually because you can use our fantastic Free Moment of Inertia Calculator to find the important properties and information about beam sections. |
Bending Moment and Shear Force Diagram Calculator
The first free, easy to use customizable Bending Moment Diagram and Shear Force Diagram Calculator for simply supported Beams